Nowadays, a number of people afflicted with diabetes mellitus is increasing from 382 million to 592 million as a forecast for a period from 2013 to 2035 [1]. In 2011, 1.4 million people around the world had died due to diabetes mellitus being the one of the 8 top causes of death [2]. Diabetes is a disease causing serious long-term influence. The most significant consequences are cardiovascular, retinal, renal and neuropathic pathologies. Determination glucose concentration in blood is an important method to diagnose the patients who are afflicted by diabetes mellitus. Conventional blood examination methods using drawn blood such as test-strip, electronic glucose meter, interstitial blood fluid and enzyme electrodes are invasive techniques leading to the risk of infection. They are also painful and uncomfortable in several situations. Noninvasive techniques were developed for glucose monitoring as well as other blood analysis. They eliminated the disadvantages of invasive methods. Many optical techniques were employed to overcome the drawback such as polarized light measurement, Raman spectroscopy, near infrared spectroscopy and others. They provide non-ionized and reduce the risk of radiation for medical applications. However, using each method depends on aims of studies such as: optical polarization provides information about structurally anisotropic tissue components: collagen and muscle fiber; optical spectra based on absorption, Raman scattering provides biochemical information because it is related to molecular; optical absorption provides contrast for functional image, etc. Moreover, there were several popular reconstruction image methods that were also applied: Diffuse Optical Tomography (DOT) with excellent contrast and good imaging depth (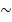 50mm), but poor resolution (
50mm), but poor resolution (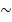 5mm) [3]; Ultrasound with excellent resolution (
5mm) [3]; Ultrasound with excellent resolution (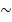 0.3mm), good imaging depth (
0.3mm), good imaging depth (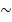 60mm), limited by poor contrast [3]; Photo Acoustic Tomography with excellent contrast, good imaging depth (
60mm), limited by poor contrast [3]; Photo Acoustic Tomography with excellent contrast, good imaging depth (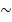 60mm) and excellent resolution (0.01mm) [3]. With the aims of precise determination targeting to glucose concentration in blood, good glucose imaging depth with excellent resolution images is required, a Photo Acoustic Tomography method can be considered. This method is based on photo acoustic effects. When the light passes a biological tissue, an acoustic wave is induced due to elastic thermal caused by interaction of photo streams with the tissues. These ultrasound waves were acquired and then reconstructed with the Time Reversal Algorithm to be an image which the pattern performs in high contrast [3]. The intensity values of the photo acoustic image presented as overlap absorption of the components, leading to imprecise measurement of distribution of each component concentration through the absorption coefficient. The Photo-Acoustic Spectroscopy method can be a solution to extract photo-acoustic spectrum of the components based on their natural frequencies from the measured complex signal. Using the spectrum analysis it is possible to calculate the precise values of the component concentration in the image pattern.
60mm) and excellent resolution (0.01mm) [3]. With the aims of precise determination targeting to glucose concentration in blood, good glucose imaging depth with excellent resolution images is required, a Photo Acoustic Tomography method can be considered. This method is based on photo acoustic effects. When the light passes a biological tissue, an acoustic wave is induced due to elastic thermal caused by interaction of photo streams with the tissues. These ultrasound waves were acquired and then reconstructed with the Time Reversal Algorithm to be an image which the pattern performs in high contrast [3]. The intensity values of the photo acoustic image presented as overlap absorption of the components, leading to imprecise measurement of distribution of each component concentration through the absorption coefficient. The Photo-Acoustic Spectroscopy method can be a solution to extract photo-acoustic spectrum of the components based on their natural frequencies from the measured complex signal. Using the spectrum analysis it is possible to calculate the precise values of the component concentration in the image pattern.
This paper presents pilot studies to assert the feasibility of Photo-Acoustic Tomography combined with Photo-Acoustic Spectroscopy applied to quantitative clinical examination as noninvasive measurement for blood analysts. For the assessment, we examined the technique in the case of glucose measurement as an example. To estimate the parameters of the practical measurement systems in the future, we firstly simulate to obtaining the results using a program that was built in Matlab environment.
Theoretical basis
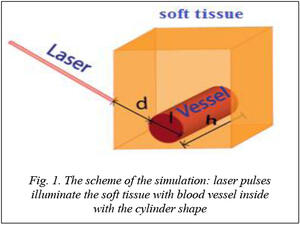 The photo-acoustic imaging is usually obtained with illumination from an optical pulses train with the duration of r(t – t) because TOF (time-of-flight) of photo-acoustic waves is used to determine the spatial information of wave sources. Assuming that, a photo-acoustic image for a blood vessel, which has been shown in figure 1, will be imaged with the condition of the light energy as
The photo-acoustic imaging is usually obtained with illumination from an optical pulses train with the duration of r(t – t) because TOF (time-of-flight) of photo-acoustic waves is used to determine the spatial information of wave sources. Assuming that, a photo-acoustic image for a blood vessel, which has been shown in figure 1, will be imaged with the condition of the light energy as
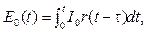 (1)
(1)
where I0 is the intensity amplitude of the optical pulses.
When the soft tissues with the absorption coefficient ast are illuminated by optical pulses, a part of light energy is absorbed and at the boundary of the vessel, the intensity amplitude of optical pulses are reduced in Beer-Lambert law [3] so that
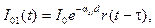 (2)
(2)
where d represents the distance from the illuminated point posed on the boundary of the biological tissue to the boundary of blood vessel. After pass through the blood vessel, the absorption energy can be written as
 (3)
(3)
with a, l as the absorption coefficient and the diameter of the blood vessel (optical path length), respectively.
Taking into account a length of time from 0 to t, Equation 3 can be rewritten as
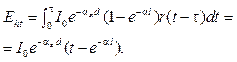 (4)
(4)
Beside it, the converted heat energy in blood vessel is also presented as
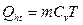 . (5)
. (5)
With m is mass of blood (Kg); T is a temperature (K); Cv is specific heat capacity (J/KgK).
Assuming that, the efficiency of conversation between absorption light energy and heat energy in blood vessel is hth. The heat energy can be presented as Qht = hthEht,
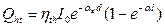 . (6)
. (6)
Insertting equation 6 into equation 5, we obtain
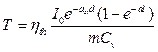 . (7)
. (7)
On the other hand, the equation of the state applied to ideal gas law is expressed by Clapeyron as [4]
PV=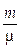 RT. (8)
RT. (8)
In which, P is pressure (Pa); V is a volume of blood (m3); T is a temperature (K); m is a mass (Kg); m is a molar mass (Kg); R is a constant 8.31 (Pa m3/mol K).
Replacing equation 7 by equation 8 with consideration of a volume unit, the pressure induced by the heat can be expressed as:
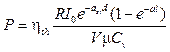 . (9)
. (9)
Equation 9 presents a relationship between the localized photo-acoustic pressure and the incident light intensity.
Takeing into account a position x inside the blood vessel at a point of time t, the photo-acoustic wave equation can be rewritten in Feynman derivation [5] as
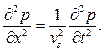 (10)
(10)
The photo-acoustic pressure p(x, t) is the solution of the wave equation [5] and presented as:
p(x, t) = P cos (wt ± kx – q), (11)
here P is amplitude of a localized photo-acoustic pressure; w angular natural frequency of the acoustic medium; 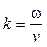 with v = 1 540 m/s wave velocity; q is an initial angular phase.
with v = 1 540 m/s wave velocity; q is an initial angular phase.
Inserting the equation 9 into equation 11, the photo-acoustic pressure function can be presented as
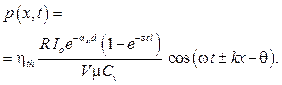 (12)
(12)
The biological tissue is structured from sophisticated components including organelles in which each component owns a natural frequency. Thus, the equation 12 can be considered as a complex acoustic wave at the position x and time t which is synthesized from the component waves generated by a single laser pulse. According to above mentioned, assuming that inside the vessel, the blood contains components whose each ith agent has a specific molar absorptivity ei, absorption coefficient ai with ai = eici (ci is concentration of the ith agent in the blood vessel), angular natural frequency wi and molar mass mi. The photo-acoustic component wave generated by each component medium is written as
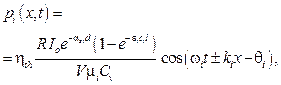 (13)
(13)
here the term 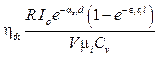 mentions to the amplitude of the each component photoacoustic wave, Pi. Under this consideration the measurement photoacoustic wave is synthesized from M component photoacoustic waves generated by the M agents in the blood vessel, equation 11 can be rewritten as
mentions to the amplitude of the each component photoacoustic wave, Pi. Under this consideration the measurement photoacoustic wave is synthesized from M component photoacoustic waves generated by the M agents in the blood vessel, equation 11 can be rewritten as
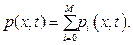 (14)
(14)
Due to the synthesis of the photo-acoustic waves, the concentrations of the agents measured through the light absorbance of the medium are superposed, then lead to the inaccuracy. To measure the agent concentration precisely, the photo-acoustic complex wave should be extracted to be individual acoustic waves based on natural frequency. For this purpose the Discrete Fourier transform can be considered and the transform equation for p(x, t) signal with N samples in a period as [6] will be
 (15)
(15)
After using the Discrete Fourier transform the amplitude Pi of each component of a photoacoustic wave is determined. The concentration of each agent is obtained as
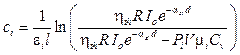 . (16)
. (16)
Figure 2 illustrates a scheme for a photo acoustic Spectroscopy method.

Simulation
The concentration of blood agents can be calculated directly from equation 16 after receiving Pi from the Fourier analysis through equation 15. However, the images showing the concentration distribution dynamically with the data of the individual photoacoustic wave should be examined to assert the feasibility for the development based on this technique toward clinical application as well as the visualization provision for the observers. For simplicity, here, we assume that the conversation between light energy and heat energy is 100 %. So, equation 12 can be rewritten as:
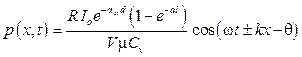 . (17)
. (17)
To simulate the scheme of the measurement, we used an aqueous mixture pattern in a blood vessel that includes 5 agents: Glucose, Urea, Albumin, 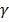 -Globulin. Some reports [3] discuss the simulation of the light propagation in biological tissues proposed under consideration of the energy transfer approximated as diffusion equation. It is called a forward problem. Due to the small acoustic scattering coefficient of the biological tissue [7], the attenuation of the photoacoustic wave is neglected. In the condition of scattering dominated media, the value of ast in equation 17 corresponding to the effective attenuation coefficient in soft tissue was estimated at 6.88 cm–1 [8, 9].
-Globulin. Some reports [3] discuss the simulation of the light propagation in biological tissues proposed under consideration of the energy transfer approximated as diffusion equation. It is called a forward problem. Due to the small acoustic scattering coefficient of the biological tissue [7], the attenuation of the photoacoustic wave is neglected. In the condition of scattering dominated media, the value of ast in equation 17 corresponding to the effective attenuation coefficient in soft tissue was estimated at 6.88 cm–1 [8, 9].
According to some reports, at the wavelength of 1 600 nm the molar absorptivity of glucose was the most different in comparison with other agents [10, 11]. Therefore, this wavelength was considered to determine precisely the glucose concentration precisely.
Table 1
The parameters of blood agents in simulation conditions including ai, mi, fi, and ei
| |
Glucose
|
Urea
|
Albumin
|
g-Globulin
|
Water
|
|
a
cm–1
|
0.01
|
1.4×10–4
|
4.18×10–3
|
4.18×10–2
|
1.34×10–3
|
|
m
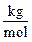
|
0.18
[12]
|
0.06
[13]
|
66.5
[14]
|
150
[15]
|
0.018
[16]
|
|
f
Hz
|
1×10–7
|
3×10–7
|
5×10–7
|
7×10–7
|
10×10–7
|
|
e

|
0.644
|
0.045
|
9.28
|
24.19
|
0.005
|
Table 2
Physical properties of the simulated samples
|
Conditions
|
Values
|
|
Thickness of mixture pattern ( l )
|
0.1 cm [11]
|
|
Effective attenuation coefficient of soft tissue
|
6.88 cm–1 [8, 9]
|
|
Initial phase angular q
|
p/4
|
|
Position x
|
0.05 cm
|
|
Specific heat capacity Cv
|
4000 (J/KgK) [3]
|
|
Velocity wave in mixture v
|
1540 m/s [3]
|
|
The effective height of vessel (h)
|
h=1 mm
|
|
The volume of blood vessel (V)
|
1.57x 10–9 m3
|
At 1 600 nm specific absorption (ai) [10, 11], molar mass (mi), molar absorptivity (ei), natural frequencies (fi) of Glucose, Urea, Albumin, g-Globulin and Water were addressed via the references [10, 11] and assumed as shown in table 1. Table 2 describes physical properties of the simulated samples. The scattering of components also can be neglected because they are too small in 1mm thickness of the mixture pattern.
Based on equation 1, a pulse train which is used as excitation light to the mixture and has duration t:(t – t), is generated from two step pulses r(t – t) = = u1(t) + u2(t) with 
u1(t) = u(t – t1), u2(t) = –u(t – t2), duration t = t2 – t1. In this simulation with optical length of 0.1 cm t was calculated as 4´10–7 s [3].
With sampling frequency 100´107 Hz we generated the waves for each component in a mixture, then they was superposed leading to a complex acoustic wave:
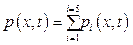 = pglucose (x, t) + purea (x, t) + + palbumin (x, t) + pg–globulin (x, t) + pwater (x, t).
= pglucose (x, t) + purea (x, t) + + palbumin (x, t) + pg–globulin (x, t) + pwater (x, t).
This wave were analyzed based on natural frequencies by Discrete Fourier transform in Matlab environment (N=10 000 samples), the component photo-acoustic waves were separated. Using peaks detection algorithm, we obtained the individual photo-acoustic pressure amplitudes of component agents. The concentration of agents is calculated as
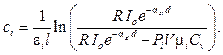 (18)
(18)
To visualize the concentration distribution, the numerical results of this simulation were assigned using a color. Each color represents each agent and a mixed color expresses a mixture.
Results and discussion
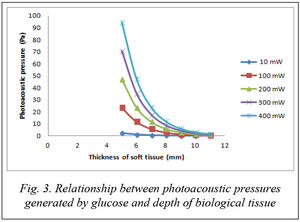 Figure 3 shows the relationship between photoacoustic pressure generated by glucose and the depth of soft tissue under excitation power of laser.
Figure 3 shows the relationship between photoacoustic pressure generated by glucose and the depth of soft tissue under excitation power of laser.
As shown in figure 3, with excitation laser power of 300 mW, at the soft tissue depth of 10 mm, the photoacoustic pressure corresponds approximately to 2.27 Pa. This pressure is available for measurement using a typical ultrasonic transducer in sensitivity of 40 nV/Pa.
Figure 4 presents square pulses with period time T equal to 10´10–7 s, duration t equal to 4´10–7 s were presented in figure 4.
When pulse train excited a mixture, complex acous- tic waves were recorded and expressed using t= (0:35) ´10–7 s as shown in figure 5.
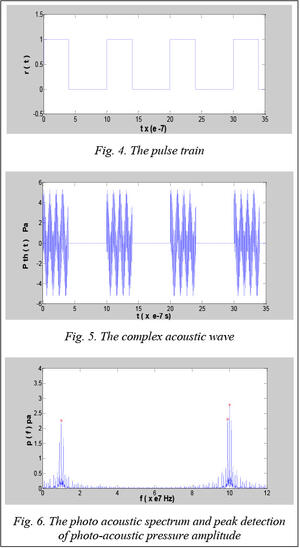 Using Fourier analysis for this complex acoustic wave, we obtained the results the spectrum of photo-acoustic pressure generated from the mixture under excitation of the light pulse train. Then we detected the peak values represented in the photo-acoustic pressure amplitudes of each component using the peak searching algorithm. The results were indicated as shown in figure 6.
Using Fourier analysis for this complex acoustic wave, we obtained the results the spectrum of photo-acoustic pressure generated from the mixture under excitation of the light pulse train. Then we detected the peak values represented in the photo-acoustic pressure amplitudes of each component using the peak searching algorithm. The results were indicated as shown in figure 6.
At frequency of 107 Hz, the photo-acoustic pressure amplitude of Glucose reached to 2.27 Pa. Using this value we calculated glucose concentration based on equation 18 to obtain the concentration value of 0.016 mol/L corresponding to 2.8 g/L.
Correspondingly, the values of the agent concentrations were assigned to intensity the components’ color in range of human physiological conditions. In normal human blood concentration ranges of Glucose [17], Urea [17], Albumin [18], g-Globulin [18] were estimated in table 3. Furthermore, with molar absorptivity of water is 0.005 and specific absorption is 1.34´10-3 cm–1 [10, 11], we also have volumetric distribution of water 0.268 mol/L corresponding to 482.4 mg/dL. A mixture was produced by dissolving from the above agents.
Figure 7 shows the color of the mixture. To separate the agents for concentration distribution observation, the color of the mixture is presented as the component’s colors which are used to synthesize the mixture color. It is easy when component agents are extracted from the mixture.
Table 3
Concentration ranges of agents in normal human blood
|
Agents
|
Concentration ranges
|
|
Glucose
|
65–105 mg/dL
|
|
Urea
|
7–18 mg/dL
|
|
Albumin
|
3200–4800 mg/dL
|
|
g-Globulin
|
500–1500 mg/dL
|
|
Water
|
482.4 mg/dL
|
Each color represents one agent: Glucose-red, Urea-pink lotus, Albumin-bone, gGlobulin-green, Wa- ter-turquoise. Brightness of colors (dark to light) is based on concentration of component agents as shown in figures 8–12.
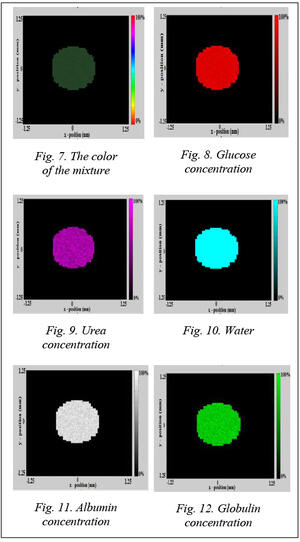 Concentration ranges of Glucose, Urea: 65–105 mg/dL, 7–18 mg/dL with 0–100 % respectively.
Concentration ranges of Glucose, Urea: 65–105 mg/dL, 7–18 mg/dL with 0–100 % respectively.
Concentration ranges of g-Globulin, Albumin: 500–1 500 mg/dL, 3 200–4 800 mg/dL with 0–100 % respectively.
Conclusion
The technique being a combination of Photo Acoustic Tomography and Photo Acoustic Spectroscopy applied to blood analysis was demonstrated using a numerical simulation. Throughout an example of glucose measurement scheme, the precise glucose concentration was extracted from an imitated blood compound including 5 different components. Under the condition of 1 mm vessel diameter and 10 mm thickness of soft tissue with the light excitation of 300 mW generated through the pulse train with its width 0.4 microseconds, the 2D cross-sectional images were reconstructed. The intensities at image point data can be used to indicate the concentration quantitatively by Fourier analysis. With the sensitivity of few pascals for the photo-acoustic pressure level and several hundreds milliwatts for the light excitation, this technique promises the developments concerning to clinical applications of blood analysis practically in the near future.
References
1. Intern/ Diabetes Foundation: Diabetes Atlas. Available at: http://www.diabetesatlas.org/ (accessed April 4, 2014).
2. Diabetes Fact sheet N°312. WHO. 2013. Available at: http://www.who.int/mediacentre/factsheets/fs312/en/ (accessed March 25, 2014).
3. Wang L.V., Wu H. Biomedical Optics: Principles and Imaging. Wiley-Interscience Publ., Hoboken, NJ, 2007.
4. Balasubramanian V. Chemistry higher secondary – first year. Tamilnadu Textbook Corp., College Road, Chennai, 2004, no. 1, Unit 10.
5. Feynman R., Leighton R.B., Sands M. The Feyman Lectures on Physics. Addison Publ., 1969, no. 1, pp. 47-1–47-8.
6. Li Tan. Digital Signal Processing Fundamentals and Applications. Academic Press, Elsevier, 2008, pp.105–152.
7. Shung K.K., Thieme G.A. Ultrasonic Scattering in Biological Tissues. CRC Press, America, 1993, 512 p.
8. Bashkatov N.A. Optical Properties of the Subcutaneous Adipose Tissue in the Spectral Range 400–2500 nm. Optics and Spectroscopy. 2005, vol. 99, iss. 5, pp. 836–842.
9. Khalil O.S. Spectroscopic and Clinical Aspects of Noninvasive Glucose Measurements. Clinical Chemistry. 1999, vol. 45, iss. 2, pp. 165–177.
10. Amerov K., Chen J., Arnold M.A. Molar Absorptivities of Glucose and Other Biological Molecules in Aqueous Solutions over the First Overtone and Combination Regions of the Near-Infrared Spectrum. Applied Spectroscopy. 2004, no. 58, pp. 1199–1201.
11. Kasemsumran S. Analytica Chimica Acta. 2004, vol. 512, pp. 223–230.
12. Brown Th.E., Eugene H., LeMay H. Chemistry The Central Science. Prentice Hall Publ., Pearson, 2011, 89 p.
13. Zumdahl S., DeCoste D.J. Chemical principles. Brooks/Cole, Davis Drive Belmont, 7th ed., 2011, pp. 865–866.
14. Strege M.A., Lagu A.L. Capillary Electrophoresis of Proteins and Peptides. Human Press, NJ, 2004, 332 p.
15. Rogers K. The components of Life from Nucleic Acids to Carbohydrats. Britanica Educational Publ., NY, 1st ed., 2011, 242 p.
16. Brown L., Holme T. Chemistry for Engineering. Cengage Learning, 2013, 608 p.
17. Shaw A.R., Mantsch H.H. Encyclopedia of Analytical Chemistry. John Wiley & Sons Ltd. Publ., Chichester, 2000, pp. 83–102.
18. Faller A., Schuenke M. The Human Body: An Introduction to Structure and Function. Georg Thieme Verlag Publ., Germany, 2004, 720 p.



 50mm), but poor resolution (
50mm), but poor resolution (
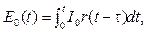 (1)
(1)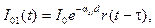 (2)
(2) (3)
(3)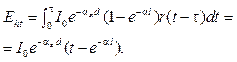 (4)
(4)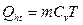 . (5)
. (5)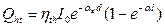 . (6)
. (6)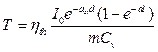 . (7)
. (7)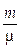 RT. (8)
RT. (8)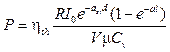 . (9)
. (9)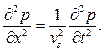 (10)
(10)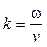 with v = 1 540 m/s wave velocity; q is an initial angular phase.
with v = 1 540 m/s wave velocity; q is an initial angular phase.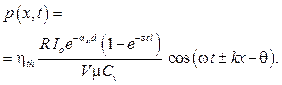 (12)
(12)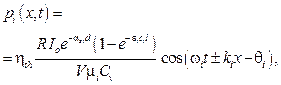 (13)
(13)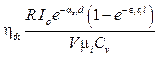 mentions to the amplitude of the each component photoacoustic wave, Pi. Under this consideration the measurement photoacoustic wave is synthesized from M component photoacoustic waves generated by the M agents in the blood vessel, equation 11 can be rewritten as
mentions to the amplitude of the each component photoacoustic wave, Pi. Under this consideration the measurement photoacoustic wave is synthesized from M component photoacoustic waves generated by the M agents in the blood vessel, equation 11 can be rewritten as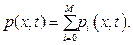 (14)
(14) (15)
(15)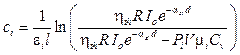 . (16)
. (16)
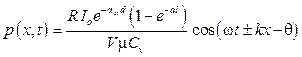 . (17)
. (17) -Globulin. Some reports [3] discuss the simulation of the light propagation in biological tissues proposed under consideration of the energy transfer approximated as diffusion equation. It is called a forward problem. Due to the small acoustic scattering coefficient of the biological tissue [7], the attenuation of the photoacoustic wave is neglected. In the condition of scattering dominated media, the value of ast in equation 17 corresponding to the effective attenuation coefficient in soft tissue was estimated at 6.88 cm–1 [8, 9].
-Globulin. Some reports [3] discuss the simulation of the light propagation in biological tissues proposed under consideration of the energy transfer approximated as diffusion equation. It is called a forward problem. Due to the small acoustic scattering coefficient of the biological tissue [7], the attenuation of the photoacoustic wave is neglected. In the condition of scattering dominated media, the value of ast in equation 17 corresponding to the effective attenuation coefficient in soft tissue was estimated at 6.88 cm–1 [8, 9].


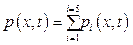 = pglucose (x, t) + purea (x, t) + + palbumin (x, t) + pg–globulin (x, t) + pwater (x, t).
= pglucose (x, t) + purea (x, t) + + palbumin (x, t) + pg–globulin (x, t) + pwater (x, t).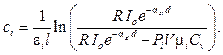 (18)
(18)


